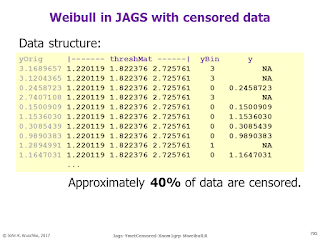
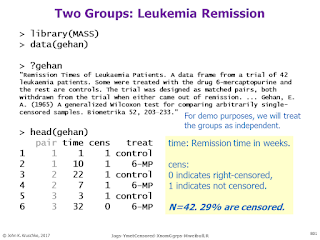
Richard Charnigo, Professor of Biostatistics, College of Public Health, University of Kentucky, has posted lecture slides online for his Bayesian course that used DBDA2. On his website he states,
"Here is a zipped folder containing my lecture slides and related materials for the “Bayesian Biostatistics” special topics course that I taught in Spring 2025. The lecture slides largely follow Prof. Kruschke’s book entitled Doing Bayesian Data Analysis, second edition, whose cover displays four puppies demonstrating Bayes’ Rule. As the special topics course had no prerequisites and was open to both undergraduate and graduate students, we covered only what Prof. Kruschke referred to as the “minimalist excursion” of his book. Still, that material was treated in some depth, and the interested student might continue with other chapters.
Permission is hereby granted to use these materials for self-study and, for faculty instructors of relevant courses, to use or adapt these materials for didactic purposes. If adapting these materials, please do include an appropriate credit (e.g., “Modified from materials prepared by Prof. Charnigo”). For faculty instructors of relevant courses, my solutions to the portfolio contributions are also available upon written request. Finally, I state my objection to using pirated intellectual property; in particular, please buy Prof. Kruschke’s book if you want to use it, or otherwise access it legally (e.g., in a library or via institutional subscription). Thank you."
Thank you, Richard, for making the materials available.
If anyone else has course materials available and would like me to post a notice here, please let me know.